
- Видео 717
- Просмотров 12 481 178
Prime Newtons
США
Добавлен 14 ноя 2019
This channel is to help College and High school students master essential math skills in order to be ready for higher mathematics . I present and solve select questions in each video.
1973 USAMO ( System of equations)
This was a little tricky to show that no other solutions were possible.
Просмотров: 3 636
Видео
First Pigeonhole Principle
Просмотров 1,7 тыс.4 часа назад
In mathematics, the pigeonhole principle states that if n items are put into m containers, with n m, then at least one container must contain more than one item.
Cubic equation from determinant
Просмотров 3,6 тыс.14 часов назад
This determinant is computed using cofactor expansion. The resulting cubic equation is then factored using synthetic division since one root is already given. Get merch here: rb.gy/cya1qk
2015 Denmark [Georg Mohr]
Просмотров 5 тыс.16 часов назад
This system of equations required showing that some equations were possible for real numbers and some where not.
A problem from Lithuania 2010
Просмотров 4,9 тыс.19 часов назад
proving an inequality is always interesting because there are 3 main strategies I adopt. I highlighted them in the video and used two of them in combination. Get merch here: rb.gy/cya1qk
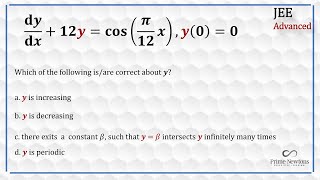
JEE Advanced 2022 #14
Просмотров 4,2 тыс.21 час назад
This problem required solving a first order linear differential equation using the method of integrating factor. The student is then required to deduce the properties of the function obtained.
Another System of Equations from Denmark
Просмотров 3,3 тыс.21 час назад
While solving some system of equations where the variiables can not be easily isolated for substtitution, it may be easier to replace any convenient combination of the variables with another variable.
System of Symmetric Equations
Просмотров 4,9 тыс.День назад
While solving some system of equations where the variiables can not be easily isolated for substtitution, it may be easier to replace any convenient combination of the variables with another variable.
Improve your u-sub
Просмотров 2,8 тыс.День назад
U-substitution ( change of variable) is the most used integration strategy in calculus. We start small, but with time, we see that it becomes more efficiant if we replace a bigger portion of the integrand the very first time. nless interrupted by another integration strategy, any time you used u-sub more than once, it's a sign you did not take enough the first time.
Regional Math Olympiad Problem
Просмотров 6 тыс.День назад
This is from the Regional Math Olympiad. The trick here is that not all polynomials are solved in terms of x. Sometimes the other unknown variable becomes the key.
Invoking the Gamma Function
Просмотров 6 тыс.День назад
This definite integral could not be evaluated using the integration techniques learned in calculus2. I showed that the problem could be modified by appropriate substitution to facilitate the use of the gamma function for its evaluation.
An exponential trig equation
Просмотров 3 тыс.14 дней назад
This was a problem from the Canadian Euclid Math Contest from 2024 . A 12 grader is expected to easily answer this question without requiring any special knowledge or reasoning.
x - 1/x² = (rad2)i , Find x^2187 - 1/x^2187.
Просмотров 3,8 тыс.14 дней назад
This was a multiple choice problem from Jee Admanced sent in by a subscriber. The required skills were basic algebra and a drop of keen observation.
2024 Canada Euclid Math Contest
Просмотров 5 тыс.14 дней назад
The main idea was to use the laws of logarithms and then some multiplication and division. It was a good problem to wreslte with for any 12th-grade student.
The Last 3 digits of sqrt( 1^3 + 2^3 + ...+ 2024^3)
Просмотров 4,6 тыс.14 дней назад
The main idea was to use the fact that the sum of n natural cubes is the square of the sum of n natural numbers. This is the video on the proof referred to in the video ruclips.net/video/VgwLVxLoLz0/видео.htmlsi=DLy8qqhh0NMOJDvE
A problem from Denmark 2006 (Georg Mohr)
Просмотров 5 тыс.14 дней назад
A problem from Denmark 2006 (Georg Mohr)
Position, Jerk, Pop and Other Derivatives of The Position Function
Просмотров 3,5 тыс.14 дней назад
Position, Jerk, Pop and Other Derivatives of The Position Function
A set Theory problem from JEE Advanced 2022
Просмотров 3,2 тыс.28 дней назад
A set Theory problem from JEE Advanced 2022
Third International Mathematics Olympiad #2
Просмотров 8 тыс.28 дней назад
Third International Mathematics Olympiad #2
Find the last digit of (1! + 2! +...+ 1982!)^1982
Просмотров 10 тыс.Месяц назад
Find the last digit of (1! 2! ... 1982!)^1982
A nice Completing the squares problem
Просмотров 4,6 тыс.Месяц назад
A nice Completing the squares problem



![2015 Denmark [Georg Mohr]](http://i.ytimg.com/vi/_Urn_phgPjE/mqdefault.jpg)


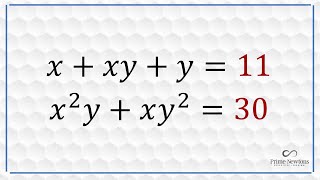

there seems to be some issue in the logic of the solution
Isnt u going to +- infinity as t approaches 0?
You are just incredible
That's the amazing topic sir I'm not actually see even in my uni lifee thanks n love from pakistan🇵🇰
Appreciate your enthusiasm ❤
Do you guys know there is something called pentation? I was searching this up and found out pentation is even larger... Brain dead
Bros cooking
really enjoyable
Note that: • the equation are cyclical • as RHS of any one equation is integer x, y and z are integers. The reasons are as follows: * if any one of x, y, and z not an integer then all equation's RHS will not be integer * if any two of x, y, and z not an integer RHS of one equation will be an integers, but those of the other ones will not. * if x, y, z are all not integer, RHS of all equations will not be integer. (x+y+z)²=x²+y²+z²+2(xy+yz+zx) 9=3+2(xy+yz+zx) xy+yz+zx=3 (x+y+z)³=x³+y³+z³ +3(x+y+z)(xy+yz+zx)-3xyz 3³=3+3³-3xyz --> xyz=1 xyz=1 implies that • x=y=z=1 • any one of x, y, and z is 1 and the other two -1 Hence (x,y,z)={(1,1,1),(1,-1,-1),(-1,1,-1), (-1,-1,1)} A simpler way to solve is to use Newton-Girard method
How i ms this man so clever
what app are you using?
🎉🎉🎉🎉
🎉🎉🎉🎉
what a nice video to watch
x+y+z=3 x^2+y^2+z^2+2xy+2xz+2yz=9 xy+xz+yz=(9-3)/2=3 x(y+z)+yz=3 x(3-x)+yz=3 x^2-3x+3=yz (x^2+y^2+z^2)(x+y+z)=9 x^3+y^3+z^3+xy(x+y)+xz(x+z)+yz(y+z)=9 xy(3-z)+xz(3-y)+yz(3-x)=6 3(xy+xz+yz)-3xyz=6 xyz=1 yz=1/x x(y+z)+yz=3 x(3-x)+1/x=3 x^3-3x^2+3x-1=0 (x-1)^3=0 x=1 xz=1/y y(x+z)+1/y=3 y=1 xy=1/z z(x+y)+1/z=3 z=1 <x,y,z>=<1,1,1>
Nice problem and video! A couple of minor issues with the proof given: 1) At 5:47 it is concluded that for positive a,b: (a^2+b^2)/2 >= ab In fact this is true for all reals a,b and indeed this is assumed and needed later at 10:27. To prove this inequality for all reals a,b: (a-b)^2 ≥ 0 <=> a^2 - 2ab + b^2 ≥ 0 <=> (a^2 + b^2)/2 ≥ ab 2) At 6:16 you square the above inequality. This step is guaranteed to be true only if the LHS and RHS are positive. However ab is not always positive and in general it is not valid to say that p>q implies p^2>q^2. For example: 1>-10 is true but 1^2>(-10)^2 is false. As it happens, in this case we are okay because the LHS and RHS are not independent values: [(a^2 + b^2)/2]^2 ≥ (ab)^2 <=> (a^4 + 2(ab)^2 + b^4) ≥ 4(ab)^2 <=> a^4 - 2(ab)^2 + b^4 ≥ 0 <=> (a^2 - b^2)^2 ≥ 0 This last line is clearly true meaning that the squared inequality holds. An alternative proof of the original inequality: 2(a^4 + (ab)^2 + b^4) ≥ 3(ba^3 + ab^3) <=> 2[(a^2 + b^2)^2 - (ab)^2] ≥ 3ab(a^2 + b^2) <=> 2[((a+b)^2 - 2ab)^2 - (ab)^2] ≥ 3ab((a+b)^2 - 2ab) <=> 2((a+b)^2 - 2ab)^2 - 2(ab)^2 ≥ 3ab(a+b)^2 - 6(ab)^2 <=> 2((a+b)^4 - 4ab(a+b)^2 + 4(ab)^2) - 2(ab)^2 ≥ 3ab(a+b)^2 - 6(ab)^2 <=> 2(a+b)^4 - 11ab(a+b)^2 + 12(ab)^2 ≥ 0 <=> (2(a+b)^2 - 3ab).((a+b)^2 - 4ab) ≥ 0 [*] (a+b)^2 - 4ab = a^2 + 2ab + b^2 - 4ab = a^2 - 2ab + b^2 = (a-b)^2 ≥ 0 2(a+b)^2 - 3ab = 2(a^2 + 2ab + b^2) - 3ab = 2(a^2 + b^2) + ab Let a=r.cos(t) and b=r.sin(t): = 2r^2(cos^2(t) + sin^2(t)) + r^2.sin(t).cos(t) = r^2(2 + sin(2t)/2) ≥ r^2(2 - 1/2) = (3/2)r^2 ≥ 0 So the 2 terms on the LHS of [*] are indeed non-negative.
I am an Indian and i am really obsessed by your teaching ....... you have that art to teach and make things clear to us . i show my gratitude respectively.❤❤❤🎉🎉😊😊😊😊 you have 1 more subscriber 🎉🎉🎉🎉
maybe 16:37 should not be "or" but be "and" consider the meaning of vieta's formula
Thanks for this amazing video 😊
Mate i'm studying for a test, yet your attitude is so fun, i'm actually starting to like linear algebra lol. Thank you man.
respect a remake keep growing your channel
Thanks for your interesting problem. Here is the way I solved it. Of course, I didn't look at your solution. Tell me, if you like mine. Greetings and keep up the good work, with sharing us challenging problems. RECALL Solve the problem with all solutions reals and complex. (i) x+y+z=3 (ii) x^2+y^2+z^2=3 (iii) x^3+y^3+z^3=3 Let's square equation (i) x+y+z=3, then (i)^2 (x+y+z)^2=3^2 x^2+y^2+z^2+2(xy+yz+zx)=3^2 and from (ii), we have 3+2(xy+yz+zx)=3^2 then (xy+yz+zx)=(3^2-3)/2=3 so (xy+yz+zx)=3 Let equation (xy+yz+zx)=3 be (iv) Let's cube equation (i) x+y+z=3, then (i)^3 (x+y+z)^3=3^3 x^3+y^3+z^3+3xy(x+y)+3yz(y+z)+3zx(z+x)+6xyz=3^3 and from (iii), we have 3+3xy(x+y)+3yz(y+z)+3zx(z+x)+6xyz=3^3 moreover from (i), we have y+z=3-x and z+x=3-y and x+y=3-z so injecting those three equalities in the above one we have 3+3xy(3-z)+3yz(3-x)+3zx(3-y)+6xyz=3^3 then 3+3^2.(xy+yz+zx)-3xyz-3xyz-3xyz+6xyz=3^3 3+3^2.(xy+yz+zx)-3xyz=3^3 and from (iv) we have 3+3^2.3-3xyz=3^3 so we have 3-3xyz=0 then xyz=1 Let equation xyz=1 be (v) From following equations (i) x+y+z=3 (iv) xy+yz+zx=3 (v) xyz=1 we recognise the symetric functions of the roots (x;y;z) of a third degree equation at^3+bt^2+ct+d=0 with the following relations between the coefficients and the roots as below (those relations being called as well as the Vieta's formulas) x+y+z=-b/a xy+yz+zx=c/a xyz=-d/a so that -b/a=3 c/a=3 -d/a=1 If we choose a=1, we have b=-3, c=3 and d=1, leading to following equation t^3-3t^2+3t-1=0 that can be written as follows (t-1)^3=0 which gives t-1=0 and finally t=1 showing that the equation has got a triple root of value 1. To conclude the system has got the only solution (x;y;z)=(1;1;1) END
7:27 gives me chill 😂
Notice that since S_1, S_2, and S_3 are functions of x + y + z, x^2 + y^2 + z^2, and x^3 + y^3 + z^3, if you have any one solution (x_0,y_0,z_0) to a system x + y + z = a, x^2 + y^2 + z^2 = b, x^3 + y^3 + z^3 = c, all of the solutions will be the permutations of (x_0,y_0,z_0) since doing what was done here will lead to (t - x_0)(t - y_0)(t - z_0) = 0 in the end.
Accelerated Girard-Newton method: t^3 -(x+y+z)t^2 +(xy+xz+yz)t -xyz=0 t^3-3t^2+3t-xyz=0 Let's replace t with x,y,z : x^3-3x^2+3x-xyz=0 (1) y^3-3y^2+3y-xyz=0 (2) z^3-3z^2+3z -xyz=0 (3) (1)+(2)+(3) x^3+y^3+z^3-3(x^2+y^2+z^2)+3(x+y+z)-3xyz=0, 3-3*3+3*3-3xyz=0, 3=3xyz xyz = 1. Historically, Girard was the first to discover the connection between symmetric polynomials and the sum of powers solutions of a polynomial equation. It doesn't always have to be Newton Prime. At least once to be Second. Never stop learning.😎
Iam 16 and i try to solve it and finally i do it😆
The optimal triangle is the isoceles triangle (a=b=c) : one can prove geometrically( by the so-called Steiner symmetrization ) that for given circumference the isoceles triangle has the largest area .This is closely related isoperymetric inequality which states for a lane domain of given circumference the circle has the largest area. If a+b+c is minimal for given area then a^2+b^2+c^2 is also minimal. This problem has interesting connections to other geometric questions.
Sorry, I mixed up equilateral and isoceles.
Input (1 | -1 2 | 4)^100 = (-515377520732011328501159929309162469708701111249 | -515377520732011329768810529537391871205404316625 1030755041464022659537621059074783742410808633250 | 1030755041464022660805271659303013143907511838626) Result True
Terimakasih🙏 Sangat jelas, walaupun saya tidak terlalu paham bahasa Inggris. Tulisan Anda sangat sangat rapi.
Man love watching your video , it's fabulous
We already heard about "Mersenne Primes".
Sytstem of equations with symmetric polynomials In fact there are special case of symmetric polynomials called power sums There are Newton-Girard formulas which allow to express power sums in terms of elementary symmetric polynomials Elementary symmetric polynomials appear in Vieta formulas To use Vieta formulas we must have elementary symmetric polynomials
I was thinking that with the cubic there can only be three solutions. (1,1,1) is a solution, found by observation. But because the equations are symmetric, this must be a triple root. So there are no other solutions.
Very cool. So asking for potential complex roots, they were hoping to mislead you forcing you to investigate further.
Amazing how easy or difficult this channel's problems get. Thanks for the help!
I agree,the difficulty changes a lot.
Beautiful explanation, really liked the solving method!
Solved in 0.1 seconds 😂...
You are explained very well! Continue to teaching!
We can manipulate the given inequality into a sort of sum of squares form. First, notice that we only need to prove the inequality for positive a and b, since when a and/or b are negative, the LHS remains the same but the RHS can only decrease or remain the same. Now, for simplicity, we can get rid of the denominators by multiplying the inequality by 6 and move all terms to LHS yielding: 2a^4 + 2b^4 + 2a^2 b^2 - 3a^3 b - 3a b^3 >= 0 This, in turn, can be represented as a (sort of) sum of squares and fourth powers as: (a - b)^4 + (a^2 - b^2)^2 + ab (a-b)^2 >= 0 The "sort of" is due to the fact that there is ab in front of the last square, but since we already justified focusing on non-negative values of a and b in the proof, we are good to go.
³2 is 16 because of 2²² which means 2²×²=2⁴ therefore making 2×2×2×2=16, that is very confusing